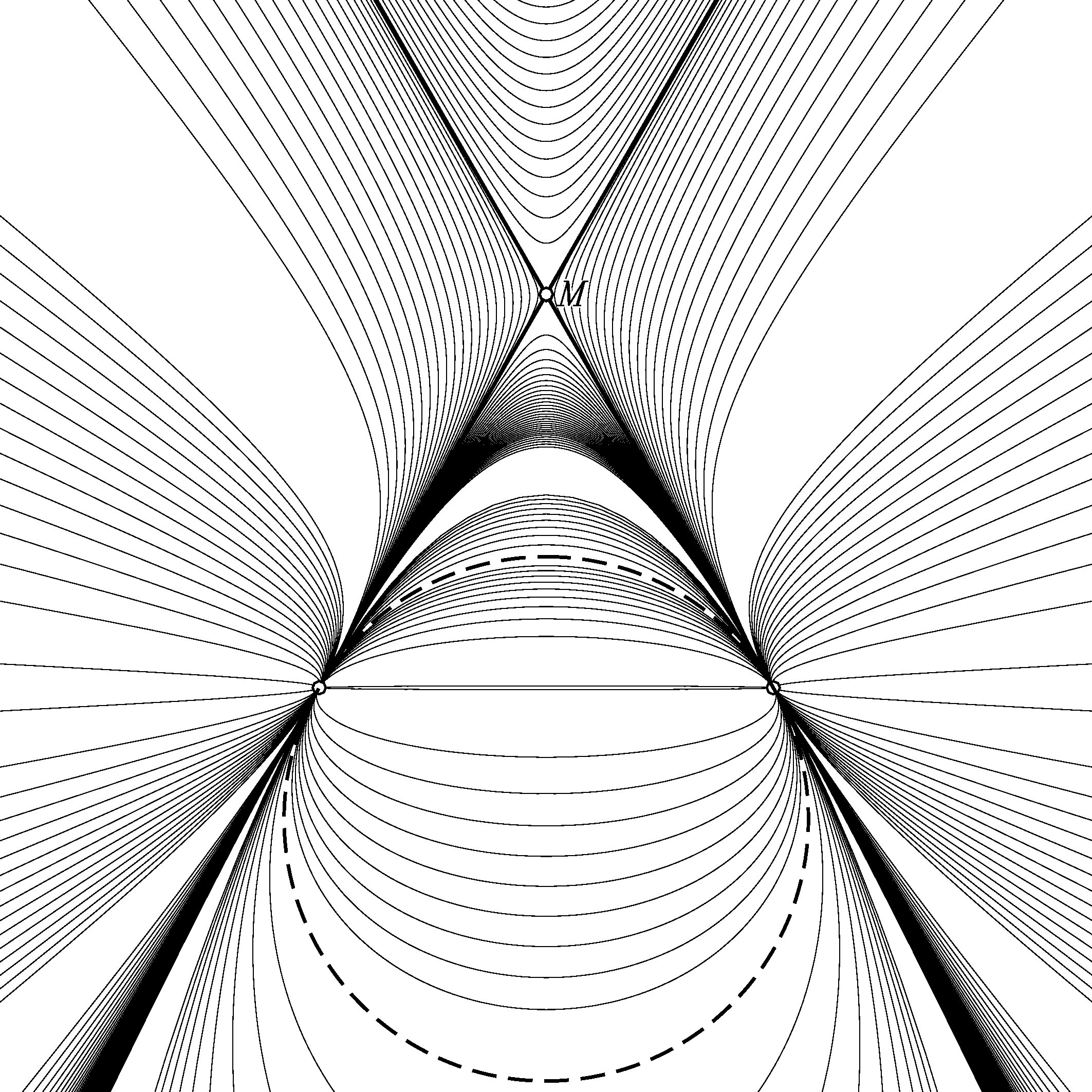
Concentric circles in deSitter geometry
We see a family of conics with two common tangents and tangent points.The intersection M of the two tangents is the center of all conics in the family: Any line through this center M has a pole common to all conics of the family. This pole lies on the polar of M, i.e. the line connecting the two contact points, of course.
When we choose one of the conics as absolute conic (which then defines the metric relations on the plane) all conics are curves of constant radius, as usual.
| Kapitel Portal |


|